How To Find X Intercept In A Quadratic Equation

How to Find the X-Intercepts and Y-Intercepts

The X-Intercepts

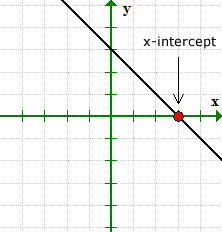
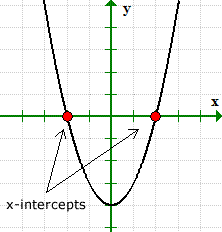
The x-intercepts are points where the graph of a function or an equation crosses or "touches" the $x$ -axis of the Cartesian Airplane. You may think of this as a point with $y$ -value of zero.

To observe the $ten$ -intercepts of an equation, allow $y = 0$ and so solve for $x$ .
In a point note, it is written equally $\left( {x,0} \right)$ .

x-intercept of a Linear Part or a Directly Line

the x-intercept of a line as shown on a graph

x-intercepts of a Quadratic Part or Parabola

the x-intercepts of a quadratic function or parabola on a graph

The Y-Intercepts

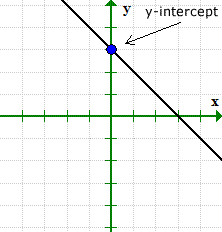
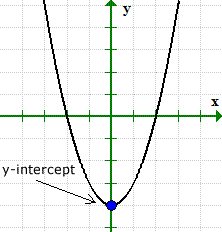
The y-intercepts are points where the graph of a function or an equation crosses or "touches" the $y$ -axis of the Cartesian Aeroplane. You lot may think of this as a point with $x$ -value of zero.

To find the $y$ -intercepts of an equation, let $ten = 0$ then solve for $y$ .
In a point notation, it is written as $\left( {0,y} \right)$ .

y-intercept of a Linear Office or a Straight Line

y-intercept of a line or linear function on a graph

y-intercept of a Quadratic Function or Parabola

y-intercept of a quadratic function illustrated on xy-axis

Examples of How to Find the x and y-intercepts of a Line, Parabola, and Circumvolve

Example 1: From the graph, describe the $10$ and $y$ -intercepts using signal notation.

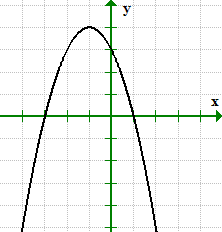
the graph of a quadratic function with x-intercepts of 1 and 3, and y-intercept of 3.

The graph crosses the $ten$ -centrality at $x= i$ and $x= 3$ , therefore, nosotros can write the $x$ -intercepts every bit points $(1,0)$ and $(-3, 0)$ .

Similarly, the graph crosses the $y$ -axis at $y=3$ . Its y-intercept can be written every bit the point $(0,iii)$ .

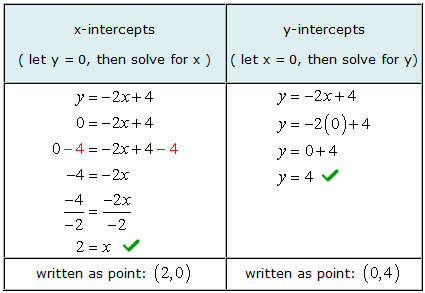
Case 2: Find the x and y-intercepts of the line $y = - 2x + 4$ .

To observe the x-intercepts algebraically, we allow $y=0$ in the equation and then solve for values of $x$ . In the same mode, to notice for $y$ -intercepts algebraically, we permit $x=0$ in the equation and then solve for $y$ .

x-intercept at (2,0), and y-intercept at (0,4)

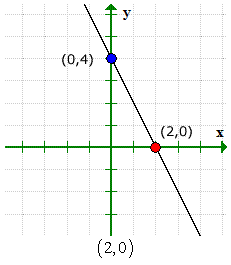
Here's the graph to verify that our answers are correct.

a line with an y-intercept at (0,4) and x-intercept at (2,0)

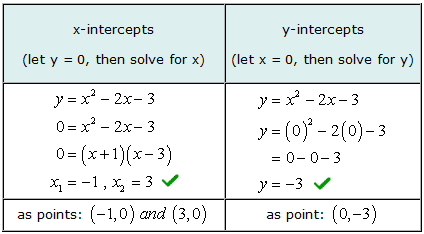
Example 3: Discover the $x$ and $y$ -intercepts of the quadratic equation $y = {ten^two} - 2x - iii$ .

The graph of this quadratic equation is a parabola. We wait information technology to take a "U" shape where it would either open or down.

To solve for the $x$ -intercept of this problem, you will factor a simple trinomial. Then you set each binomial factor equal to nothing and solve for $ten$ .

x-intercepts at (-1,0) and (3,0); y-intercept at (0,-3)

Our solved values for both $x$ and $y$ -intercepts match with the graphical solution.

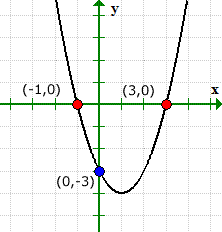
the graph of a quadratic function with x-intercepts at (-1,0) and (3,0), and y-intercept at (0,-3)

Instance four: Observe the $x$ and $y$ -intercepts of the quadratic equation $y = 3{x^2} + 1$ .

This is an example where the graph of the equation has a y-intercept but without an $x$ -intercept.

Allow'south find the $y$ -intercept first because it's extremely like shooting fish in a barrel! Plug in $ten = 0$ then solve for $y$ .

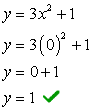
Now for the $x$ -intercept. Plug in $y = 0$ , and solve for $x$ .

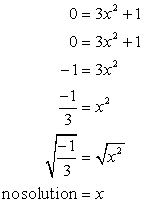
The foursquare root of a negative number is imaginary. This suggests that this equation does not have an $ten$ -intercept!

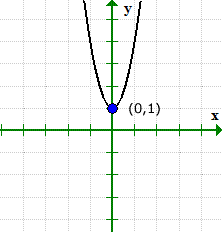
The graph can verify what'due south going on. Notice that the graph crossed the $y$ -centrality at $(0,i)$ , just never did with the $x$ -axis.

the graph of y=3x^2+1 doesn't cross or touch the x-axis

Example 5: Discover the 10 and y intercepts of the circumvolve ${\left( {10 + 4} \right)^two} + {\left( {y + ii} \right)^2} = viii$ .

This is a proficient example to illustrate that information technology is possible for the graph of an equation to have $x$ -intercepts but without $y$ -intercepts.

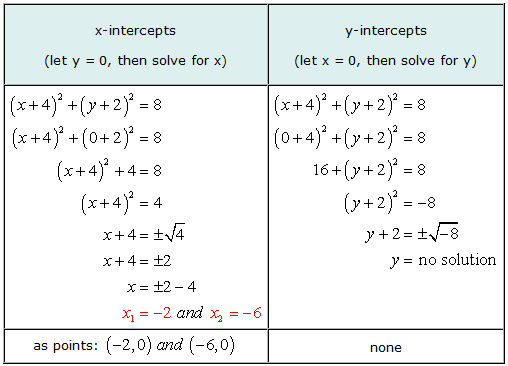
the x-intercepts of a circle are (-2,0) and (-6,0)

When solving for $y$ , we arrived at the situation of trying to get the square root of a negative number. The reply is imaginary, thus, no solution. That means the equation doesn't take whatever $y$ -intercepts.

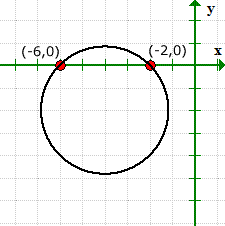
The graph verifies that we are right for the values of our $x$ -intercepts, and information technology has no $y$ -intercepts.

a circle with x-intercepts at (-6,0) and (-2,0)

Source: https://www.chilimath.com/lessons/intermediate-algebra/finding-x-y-intercepts/

Posted by: coatesperis1986.blogspot.com

How To Find X Intercept In A Quadratic Equation

How to Find the X-Intercepts and Y-Intercepts

The X-Intercepts

The Y-Intercepts

Examples of How to Find the x and y-intercepts of a Line, Parabola, and Circumvolve

0 Response to "How To Find X Intercept In A Quadratic Equation"

Post a Comment

Iklan Atas Artikel

Iklan Tengah Artikel 1

Iklan Tengah Artikel 2

Iklan Bawah Artikel